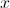Atividades Educativas

Atividades Para Imprimir

Exercícios de Matemática

Jogos de Matematica

Exames de Matemática

Estudo Função - Monotonia e Extremos Relativos - Exercícios 11º Ano - Exercício 3

Exercícios de Matemática

Estudo de uma função quanto à monotonia e existência de extremos relativos

Exercício 3

Considere a função , definida por:

$f(x) = {x^3} - 3{x^2} - 9x + 4$

Estude a função quanto à monotonia e existência de extremos relativos.

Resolução dos exercícios de Matemática:

Derivada da função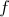

${f^'}(x) = 3{x^2} - 3(2x) - 9 + 0 = 3{x^2} - 6x - 9$

Determinar os zeros da função derivada

${f^'}(x) = 0 \Leftrightarrow 3{x^2} - 6x - 9 = 0 \Leftrightarrow x = \frac{{6 \pm \sqrt {{{( - 6)}^2} - 4(3)( - 9)} }}{{2 \times 3}} \Leftrightarrow$

$\Leftrightarrow x = \frac{{6 \pm \sqrt {36 + 108} }}{6} \Leftrightarrow x = \frac{{6 \pm 12}}{6} \Leftrightarrow$

$\Leftrightarrow x = \frac{{6 + 12}}{6} \vee x = \frac{{6 - 12}}{6} \Leftrightarrow x = 3 \vee x = - 1$

	$- \infty$	-1		3	$+ \infty$
${f^'}$	+	0	-	0	+
	$\nearrow$	M	$\searrow$	m	$\nearrow$

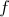é estritamente crescente em $\left] { - \infty ; - 1} \right[$ e $\left] {3; + \infty } \right[$ .

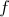é estritamente decrescente em $\left] { - 1;3} \right[$

$f( - 1) = {( - 1)^3} - 3{( - 1)^2} - 9( - 1) + 4 = - 1 - 3 + 9 + 4 = 9$

9 é máximo relativo (-1 é o maximizante)

$f(3) = {3^3} - 3 \times {3^2} - 9 \times 3 + 4 = 27 - 27 - 27 + 4 = - 23$

-23 é mínimo relativo (3 é o minimizante).

Atividade de Matemática

Atividades Educativas

Atividades Para Imprimir

Exercícios de Matemática

Exames de Matemática

Jogos de Matematica

Atividades Educativas

Atividades Para Imprimir

Exercícios de Matemática

Jogos de Matematica

Exames de Matemática

Estudo Função - Monotonia e Extremos Relativos - Exercícios 11º Ano - Exercício 3

Exercícios de Matemática

Estudo de uma função quanto à monotonia e existência de extremos relativos

Atividades Relacionadas