Resolução do exercício de matemática:
Solução: (A)
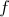 é contínua em
é contínua em ![\left] { - \infty ,a} \right[ \left] { - \infty ,a} \right[](/images/jlatex/517078a5164bf2265bd8979dea8d2cb0.gif) e em
e em ![\left] {a, + \infty } \right[ \left] {a, + \infty } \right[](/images/jlatex/846a33f1391e2eb8301e76cf8b133571.gif) .
.
![\mathop {\lim }\limits_{x \to {a^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {a^ - }} \left[ {{{\log }_3}\left( { - x - \frac{1}{3}} \right)} \right] = {\log _3}\left( { - a - \frac{1}{3}} \right) \mathop {\lim }\limits_{x \to {a^ - }} f\left( x \right) = \mathop {\lim }\limits_{x \to {a^ - }} \left[ {{{\log }_3}\left( { - x - \frac{1}{3}} \right)} \right] = {\log _3}\left( { - a - \frac{1}{3}} \right)](/images/jlatex/0146408818ddda2c3b88b72fc78b7264.gif)
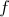 é contínua para
é contínua para  se:
se:


5. Na Figura 1, está representada, num referencial o.n. 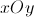 ,
,
 parte do gráfico de uma função
parte do gráfico de uma função 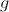 , de domínio
, de domínio  ,
,
com  .
.
Para esse valor de 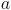 , a função
, a função 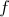 , contínua em
, contínua em 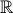 ,
,
é definida por:
 .
.
Qual é o valor de 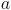 ?
?
(A) 
(B) 
(C) 
(D) 